All rights reserved.
Not long ago, Klosterneuburg near Vienna was only known for its magnificent 900-year-old monastery and fertile vineyards on the hills. Since 2009, it has become the home of a brand-new scientific institute, the Institute of Science and Technology Austria (ISTA). This bold initiative from the Austrian government aims to foster research and graduate education in the natural sciences. While so young and with 68 research groups comparably small, ISTA already ranked third in the normalized Nature Index amongst the world’s best research institutions. How did an institute located at the edge of the tranquil Vienna Woods achieve this distinction?
The formula for world-class research
ISTA was founded based on a set of principles distilled from the most successful governance systems and ideas in the world of research institutions. In fact, Professor Haim Harari, former president of the Weizmann Institute of Science, played a pivotal role in applying proven methods to the founding of ISTA. First President of ISTA is Professor Thomas A. Henzinger, a leading computer scientist with roots at the University of California in Berkeley, USA, and the EPFL in Lausanne, Switzerland. He shaped the Institute into what has become a model of academic institution to emulate.
Lab Buildings East (left) and West (right). The Institute of Science and Technology Austria (ISTA) offers state-of-the-art research facilities and scientific support units in all major disciplines. © Peter Rigaud/ISTA
©All rights reserved.
Leading a world-class research institution. The management of ISTA consists of (from left to right) Vice President for Science Education Gaia Novarino, Managing Director Georg Schneider, President Thomas A. Henzinger, Executive Vice President Michael Sixt, Dean of the Graduate School Eva Benková, and Vice President for Technology Transfer Bernd Bickel. © Peter Rigaud/ISTA
©All rights reserved.
All researchers at ISTA pursue their interests without constraints or predefined research topics. The Institute does not allocate a predetermined number of positions to different disciplines; excellence and promising research are the exclusive hiring criteria for all scientists at ISTA. Groups are supported by state-of-the-art infrastructure and scientific service units. Professors are mostly hired early in their careers on a tenure track system, usually between three to eight years after their PhD. This system provides them scientific independence and a career perspective. Since their basic research may lead to unforeseen but useful discoveries and applications, intellectual property and technology transfer are also important objectives of the Institute. The technology park adjacent to the Institute, IST Park, has developed rapidly since opening in 2019 and is already home to ten companies, including three start-ups that are based partly on Institute research.
To foster a creative atmosphere at ISTA, separating organizational structures such as faculties and scientific departments are avoided. Also, the Institute brings together researchers from all major scientific disciplines. Their communication and collaboration across fields is actively encouraged, though not mandatory – the fact that some principal areas of mathematics do not have a direct interdisciplinary component is fully recognized.
Another core mission of ISTA is science education. The Institute sees itself as a place of inquiry and reason that translates scientific practice and its results for the general public via a diverse science outreach program. Furthermore, it is a PhD-granting institution that offers fully funded PhD positions to highly qualified candidates with a bachelor’s or master’s degree in the natural sciences. Training the next generation of scientific leaders is done on graduate level, which favors advanced and research-related teaching.
Nick Barton: Mathematical Models of Evolution
Tim Browning: Analytic Number Theory and its Interfaces
Herbert Edelsbrunner: Algorithms, Computational Geometry, and Computational Topology
László Erdős: Mathematics of Disordered Quantum Systems and Matrices
Julian Fischer: Theory of Partial Differential Equations, Applied and Numerical Analysis
Tamás Hausel: Geometry and its Interfaces
Vadim Kaloshin: Dynamical Systems, Celestial Mechanics, and Spectral Rigidity
Matthew Kwan: Combinatorics and Probability
Jan Maas: Stochastic Analysis
Robert Seiringer: Mathematical Physics
Uli Wagner: Discrete and Computational Geometry and Topology
ISTA’s mathematics groups
A growing community
From the beginning, ISTA has recruited around five professors per year. From more than 15,000 applications, 68 professors are currently under contract. In the fall of 2021, the government committed another 3.3 billion Euro to secure further growth of the Institute for the next 15 years. By 2026, ISTA will reach 90 research groups, and then up to 150 groups by 2036. This growth trajectory enables it to transcend its status as a small institute and to become a substantial European hub in the global network of frontier science. New laboratory buildings, research facilities, and a visitor center are under construction and further ones are in planning to meet the needs of a growing and diversifying community.
The clear decision towards growth is based on the scientists’ achievements since the founding of the Institute. This encompasses more than 3,600 scientific publications, numerous memberships in prestigious academies and societies, and a list of renowned awards for example in mathematics: the Leonard Eisenbud Prize from the American Mathematical Society and the Erwin Schrödinger Prize from the Austrian Academy of Sciences to László Erdős, the Promotion Prize of the Austrian Mathematical Society to Julian Fischer, and twice the Ferran Sunyer i Balaguer Prize to Tim Browning.
So far 54 European Research Council (ERC) grants have been awarded to 45 professors. In mathematics, ten ERC grants for eleven research groups constitute a considerable portion of the total grant sum of 22.7 million Euro for mathematicians at ISTA. Among the graduates and alumni from mathematical groups, there are personalities like Phan Thành Nam, professor of analysis and mathematical physics at the Ludwig Maximilian University (LMU) in Munich, Germany, and Tanya Kaushal Srivastava, assistant professor of algebraic geometry at the Indian Institute of Technology (IIT) Gandhinagar.
PhD graduate Laura Schmid. Her research in computer-aided verification and game theory supervised by professor Krishnendu Chatterjee lies at the cross-section of mathematics, evolution, and computer science. © Peter Rigaud/ISTA
©All rights reserved.
Becoming a mathematician at ISTA
Two of the eleven mathematics groups began their work last year. Since hiring is done on an institute-wide level, attracting many excellent candidates in mathematics is key to maintaining its presence at ISTA. While the hiring concept is open to every direction in mathematics, ISTA strives for a healthy balance.
“Looking at the mathematics groups here, I can roughly identify three areas,” says Robert Seiringer, professor and area chair for mathematics and physics. “Five groups are working at the cross-section of mathematical physics and analysis, three groups are concerned with combinatorics and discrete/computational topology, and two groups work on algebra and number theory.” He hopes to hire colleagues in other key fields such as geometry and statistics. The envisioned development plan lays out a mosaic of mathematical expertise, where every new group – much like a tessera – feels connected to at least one existing group without duplicating it adding to a full display of cutting-edge mathematics.
The newly opened Sunstone Building. It provides space for first-year students, hosts the Institute’s library, a nuclear magnetic resonance facility, and state-of-the-art laboratories for fundamental research in chemistry and materials. Further expansion of the campus is in construction and planning. © Peter Rigaud/ISTA
©All rights reserved.
Out of the present 534 scientists 280 are PhD students, 29 of which are currently working in mathematics groups. The graduate school follows the US admission pattern: ISTA admits students once a year, with a mid-January application deadline. Students are provided with a generous fellowship for the whole duration of their studies, assuming satisfactory progress. Their first year is defined by three to five rotations, where students experience different groups in various fields until they choose an affiliation for their PhD.
The historic central building of ISTA. A place of curiosity-driven inquiry and excellent science at the borders of the peaceful Vienna Woods. © ISTA
©All rights reserved.
Postdoctoral programs are centrally financed by the ISTA Fellowship; applications are solicited with deadlines of March and September. Once again, candidates compete across disciplines for admissions. Other possibilities for fully funded, two-year postdoctoral positions are the twelve IST-BRIDGE fellowships every six months until November 2023; or the two annual NOMIS fellowships, backed by the NOMIS Foundation to advance interdisciplinary research. Brazilian mathematician Gonçalo-Oliveira, for instance, embodies the outstanding talent which ISTA and the NOMIS Foundation strive to inspire. He works at the intersection of Tamás Hausel’s and László Erdős’ groups.
For long-term visitors, there is a program with partial financing for periods of three to twelve months. Finally, ISTA offers an internship program – the ISTernship – for talented and enthusiastic undergraduate students, who would like to spend eight to twelve weeks at the Institute during the summer. A more detailed description of these opportunities may be found on the ISTA website www.ista.ac.at.
Recent research highlights in mathematics
Three recent highlights give insight into the current mathematical activities at ISTA, each exemplifying one strand of current research: algebraic geometry, mathematical physics, and number theory.
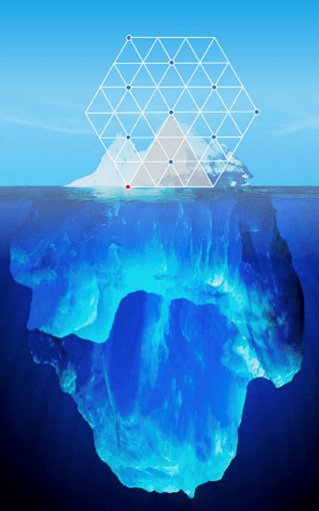
Mathematics from tip to icy toe. Professor Tamás Hausel investigates the weight diagrams in the nilpotent cone to gain knowledge about the whole underlying representation theory of Lie groups. © ISTA/Ruslan Tagiyev
©All rights reserved.
The tip of the mathematical iceberg
Mathematics may strike you as less adventurous than a polar expedition, but the exquisiteness of this conquered abstract iceberg could change your mind. The pioneers of this quest, ISTA-professor Tamás Hausel and Oxford scholar Nigel Hitchin, collaborated at the intersection of differential and algebraic geometry, connecting the distant fields of physics and number theory.
The analogy with a floating iceberg shows the significance of their mathematical expedition. The iceberg is attached to a Lie group: Most of its characteristics lie hidden beneath the surface. Down there, the interesting useful properties reside. Hausel’s and Hitchin’s elegant construction uses an abstract mathematical object from the Lie group in question, a so-called nilpotent cone of Higgs bundles. The nilpotent cone refers to the iceberg. Fortunately, the tip of its structure is completely understandable in terms of weight diagrams, which serve as visual representations of the characteristic notions of the Lie group. From the tip, they can infer knowledge about the mysterious bottom, and may even reconstruct the whole representation theory of Lie groups from it [2 T. Hausel and N. Hitchin, Very stable Higgs bundles, equivariant multiplicity and mirror symmetry. Invent. Math., DOI 10.1007/s00222-021-01093-7 (2022) ].
Unifying definitions of jellium
Jellium, or the homogeneous electron gas, is a fundamental system in quantum physics and chemistry. For instance, it is used for describing the deep interiors of white dwarfs and the valence electrons in alkaline metals. It was originally defined as an infinite gas of electrons in a positively charged uniform background, and the thermodynamic limit of the system was established rigorously. Another system, the uniform electron gas, is similar, but there is no background and the electron density is constant everywhere. In yet another model, electrons interact with periodic images of themselves.
It was conjectured in the 1980s that the ground state energies of these three systems coincide in the thermodynamic limit, but rigorously establishing this remained elusive – until now. Using a novel “floating crystal” trial state, Professor Robert Seiringer and his co-authors were able to show that these three systems are equivalent. Their argument involves “melting” a layer of crystal close to the boundary, then replacing it by an incompressible fluid. This allowed them to compensate for the charge fluctuations that occur at the system boundaries [3 M. Lewin, E. H. Lieb and R. Seiringer, Floating Wigner crystal with no boundary charge fluctuations. Phys. Rev. Lett. B 100, 035127 (2019) ].
When is necessary sufficient?
Polynomials with integer coefficients and their integer solutions have long been studied and continue to fascinate researchers up to this day. Moreover, the very existence of solutions is an important part of this: In 1900, Hilbert famously challenged researchers to design an algorithm that could say whether a polynomial equation with integer coefficients has an integer solution. We now know that in general this is not the case. However, we can say that if integer solutions exist, two conditions must hold, one having to do with solutions that are real numbers, the other with the divisibility of solutions by integers. A central conjecture in number theory suggested that if the number of variables (call this number ) of a polynomial is greater than the degree , then passing these two tests almost always means there exists an integer solution. In 2020, Professor Tim Browning and his coauthors proved the conjecture to be true in all cases except when and , a family known as cubic surfaces. Their result provides the key to creating an algorithm – of the kind Hilbert asked for – for nearly all polynomials whose number of variables is greater than their degree [1 T. Browning, P. Le Boudec and W. Sawin, The Hasse principle for random Fano hypersurfaces, preprint, arXiv:2006.02356 (2020) ].
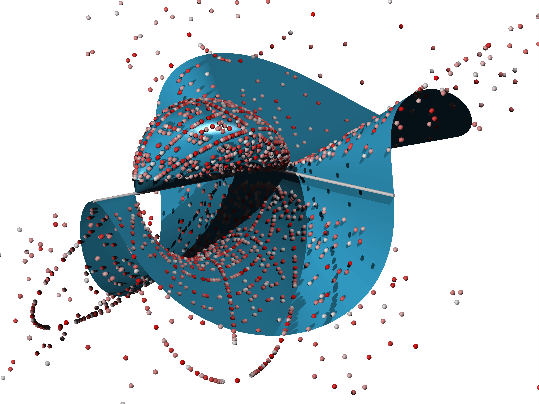
The cubic surface . It passes the two tests and has numerous integer solutions (red dots), but the conjecture remains open for cubic equations in general. © Ulrich Derenthal/Leibniz University Hannover
©All rights reserved.
References
- T. Browning, P. Le Boudec and W. Sawin, The Hasse principle for random Fano hypersurfaces, preprint, arXiv:2006.02356 (2020)
- T. Hausel and N. Hitchin, Very stable Higgs bundles, equivariant multiplicity and mirror symmetry. Invent. Math., DOI 10.1007/s00222-021-01093-7 (2022)
- M. Lewin, E. H. Lieb and R. Seiringer, Floating Wigner crystal with no boundary charge fluctuations. Phys. Rev. Lett. B 100, 035127 (2019)
Cite this article
Florian Schlederer, Mathematics at the Institute of Science and Technology Austria (ISTA). Eur. Math. Soc. Mag. 124 (2022), pp. 33–37
DOI 10.4171/MAG/82