Claire Voisin (France, 1962) is considered one of the most important experts in algebraic geometry of our time and one of the most relevant mathematical personalities of the international mathematical scene.
This interview took place in November 2023. By the end of January 2024, Claire Voisin was awarded the Crafoord Prize in Mathematics, which is granted by the Royal Academy of Sciences of Sweden and the Crafoord Foundation (Lund, Sweden) to distinguish scientific areas not included in the Nobel Prize categories. As soon as we heard about this exciting piece of news, we asked her permission to append some extra questions at the end of the interview. To get to know her better, we answered with the text below to the natural question: ultimately, who is Claire Voisin?
Claire Voisin got her PhD at the Université Paris-Sud, Orsay in 1986. Alongside her career as a researcher of the CNRS (Centre national de la recherche scientifique), she worked at the Paris-Sud University, Orsay, at the Institut de mathématiques de Jussieu (IMJ), at the Institut des Hautes Études Scientifiques (IHÉS, Bures-sur-Yvette), at the École Polytechnique (Palaiseau) and, more recently, she held the chair of algebraic geometry at the Collège de France, thus becoming the first woman to hold such an honorable position. At present, she is directrice de recherche (CNRS) at IMJ-PRG.
Claire Voisin approached and solved problems with a long history. Her name is undoubtedly connected with famous conjectures such as Kodaira’s conjecture, Hodge’s conjecture, Green’s conjecture and mirror symmetry. She was awarded several prizes among which we highlight the Shaw Prize (2017), known as the eastern Nobel Prize. We also highlight, among others, the European Mathematical Society Prize (1992), the Servant prize of the Académie des Sciences (1996), the Ruth Lyttle Satter Prize in mathematics (2007), the Clay Research Award (2008), the CNRS Gold Medal (2016), and, more recently, the L’Oréal-UNESCO Women in Science Award (2019). She was an invited speaker at the International Congress of Mathematicians (ICM) in 1994. In recognition of her contribution to science, she is a member of several scientific societies, including the Académie des Sciences, the German National Academy of Sciences Leopoldina, the Royal Society of London, the National Academy of Sciences (US) and the American Academy of Arts and Sciences.
Claire Voisin in her home-office in 2004 (courtesy of Claire Voisin).
🅭🅯 CC BY 4.0Ana Isabel Mendes / Teresa Monteiro Fernandes: Claire, we are deeply impressed to learn that you grew up in a family of 12 sisters and brothers. It is hard to imagine how your parents organized the family life and how you managed to have the quiet to become the person you are now.
Claire Voisin: It was not exactly as you say. In practice, the 12 of us never lived at home at the same time, because the family grew up with 22 years between the eldest to the youngest (the last one was born in 1968). My elder sisters left early, and I am the tenth. In my first memories, there were only five or six siblings at home. The worst was not to have a room of my own. The house was big, but the rooms were not many, however large.
[AM/TMF]: They were overcrowded…
CV: In this respect I recall with much sympathy the writer Virginia Woolf who wrote about this need for private space (A Room of One’s Own) as a condition for liberty and creativity. I had a room of my own only by the end of high school, of which I have excellent memories.
Nowadays, I have a large apartment in Paris which I enjoy very much and where I feel relaxed.
[AM/TMF]: Besides the quiet as a condition for creativity, what you achieved is so impressive that we wonder if it originated somewhere in your childhood, possibly triggered by family incentives. How were your parents?
CV: My parents were born in 1917 (my father) and 1922 (my mother). My mother had already turned 40 when I was born. With such an offspring, they were not particularly concerned about encouraging their children. However, in 1972 my father (an engineer) became unemployed. On one side it was dramatic, but on the other side this allowed him to devote time to my mathematical education. This was possible because most of my elder brothers and sisters had already left home, and my next brother had no interest in mathematics. My father had an extensive mathematical culture which he transmitted to me (geometry of triangles, some infinitesimal calculus, ellipse equation) in the old manner, since his education took place before World War II. Later, I went through a phase of criticism because at school the approach was completely different, the so-called modern mathematics, for instance, set theory, axioms, abstract operations, writing numbers in base 2 [laughs]… still the baggage learned with my father was excellent.
Another detail: my elder brother (10 years older) went to Paris to continue his studies and he left me a book on algebra, which I started studying. It was lucky.
[AM/TMF]: So, you started by yourself…, but we can say that your father influenced you.
On the occasion of the Shaw Prize, you also mentioned in an interview that the spirit of the sixties had a lot of weight on the family atmosphere. Could you be more precise?
Claire (third from the bottom left) with her family in 1966 (courtesy of Claire Voisin).
🅭🅯 CC BY 4.0CV: It was not really the sixties, but the end of the sixties, the Beatles were already the past. My parents were very intellectual, they were strongly concerned by international politics. Around 1968, we talked at table about themes like the death of Martin Luther King.
In such a way that my younger brother was named Martin. They worried much about the problems of the so-called third world: hunger, lack of freedom, etc.
On the other side, my elder brother, who was 16 at that time, was very present in the discussions because he participated in the public unrest.
My parents often talked about the philosophers of the Collège de France, about Sartre and de Beauvoir. But what they worried most about was moral implications, hence their interest in Martin Luther King Jr., but also in Abbé Pierre. Did you hear about him? He was a priest dedicated to the fight against poverty. My parents followed closely these themes and I listened to them as well as to the points of view of my elder brothers who were politically active. It was a way of drawing their attention…
[AM/TMF]: Had this social concern had an influence on you? Or had it the opposite result (as children sometimes react by opposition to their parents)?
CV: My parents were very intense, they worried about the major world problems. There was no place for individual attention. Somehow, we had no right to complain in face of the evils of the world. We were not supposed to be important. This was not a good thing. There was a total lack of ambition for us, in view of some more important things. I was lucky to escape from this way totally devoid of ambition.
This vision was excessive in face of individual work. Mathematics was for me a way of drawing my father’s attention, by contrast.
[AM/TMF]: In the seventies, in Paris, it was usual for scientists and not scientists to attend the seminars of the Collège de France given by great thinkers of the time, such as Michel Foucault. Does this habit continue?
CV: No, much less, that habit was lost. None of the present professors of the Collège de France has an influence like that of the philosophers of those years. In May of 1968 there was a mix and sharing of different forms of thinking that no longer exists.
[AM/TMF]: We read that mathematics was not your first early choice. How did you make your choice?
CV: I considered all possibilities. The director of my school advised me to enroll in the “Classes préparatoires” of the Lycée Louis-le-Grand, which I have attended from 17 to 19 years old. It was an excellent decision. The teaching was very good. I was good at mathematics, but I did not see its depth. It was like a game, but I don’t like games, I am a very serious person. I also remember having excellent literature teachers in high school.
I learned solid mathematics, but it was like a finite set of rules, one did some calculations… I was missing something; it was too practical… Of course, practice is good to understand definitions.
[AM/TMF]: Deep down, do you consider that mathematics at school is taught as a set of rules with which you must play, but there is no place left to create new things, to be creative?
CV: It was when doing research that I started taking mathematics more seriously. I was in front of something very promising, very serious. I understood then that there was so much to discover, so much we don’t know! It is not just a game at all.
[AM/TMF]: It is like lifting a veil. There is a world to be discovered. But for that one needs background…
CV: When I was about 21, I wondered whether I should study philosophy. It was at a time when I didn’t yet know what it was to do mathematics. Nowadays, I don’t regret leaving philosophy.
[AM/TMF]: Claire, you said in an interview something like “I am nostalgic for the time when my life was centered at home and shared between research and my children’s education.” For what reason?
CV: I love my children and still more since they are adults. Now that they left home, my life is very busy, I travel more, I meet more people, I participate in more commissions. In the past, my life was more concentrated: at home. I did mathematics at home, I traveled less that maybe I regret, but I consider that traveling around the world and discussing with people is more of a distraction than anything else. What did it bring to me? That the most important thing for me is to stay inside myself. Personally, I don’t think it is essential to interact with many people. So, what I meant is that, up to a certain moment, I had only mathematics and my family life. The contrast of mathematics with family life was crucial. Mathematics was my space, just mine.
[AM/TMF]: In Portugal, at the university, one has too many teaching duties, which overloads mothers’ lives. Childcare falls much upon the mother.
CV: In France, although not so as 30 years ago, the support for childhood is very good. School is mandatory at the age of 3, and before that daycare is available from 3–4 months old. I had a lot of help! What was complicated was to convince people of the importance of doing mathematics at home. Now and then I tried to have one of my children with me, but it was too hard.
[AM/TMF]: So, there is nothing to regret…
CV: Nowadays, I have more time, but I don’t do more… before I only lived for the central aspects, in a focused way.
Claire and her children in the garden, in 1998 (courtesy of Claire Voisin).
🅭🅯 CC BY 4.0[AM/TMF]: Claire, you know that you are an example of how maternity is not a prevention for great success in research. Apart from sharing tasks with your husband, himself a well-known mathematician, have you any secret of how to make the most of your time?
CV: Mathematics was my space. I started again quickly after each birth. Sometimes I had to fight for my mathematical life… Because it is my way of expressing myself.
It was not so hard because our life was simple, but we were also well organized. We lived in a small town not needing a car, everything was close and simple.
[AM/TMF]: We are aware that painting and music have a special place in your life. Let us begin with painting.
CV: When I was 19, 20, until 22 years old, I painted a lot. But just as a complement to mathematics. My social life was practically empty. It is not in my nature to relate with other people.
[AM/TMF]: However, you met your husband!
CV: [laughs] Meeting my husband was almost a miracle; at the time he was a professor in Orsay where I was preparing my PhD, but we did not meet there. It was lucky. I was very lucky. In fact, throughout life, I never had to take great decisions, things just happened.
[AM/TMF]: Now you have a greater social life, after so many prizes and the fame after so many outstanding results you achieved.
Changing the subject, one of us has the pretension of painting… Could we see one of your favorite works and include it in the published version of this interview?
CV: Of course, I am going to show you my painting that I consider the best. I like it very much, and so I keep it. I painted it when I was very young. Now I am more focused on drawing. I draw small things like trees, simple things.
In the past, with my children, we used to do lots of things, modeling… None of them were very talented. Correction, two of them were. Those were very nice moments! I also enjoyed educating and making activities, but not for playing pretend.
At the IHÉS in 2007 (courtesy of Claire Voisin).
🅭🅯 CC BY 4.0[AM/TMF]: Did you help your children in mathematics?
CV: It was not an easy task. At first, I tried to teach them, but my approach was too abstract. 9- and 10-year-old children learn easily, but they don’t have a great capacity for abstraction. I ended up teaching simple things like systems of two equations with two unknowns, for instance. Two of my 5 children are mathematicians: when they were teenagers it became hard to discuss mathematics with them, and the older they grew the worse. They wished to discover by themselves, maybe I was too fast. My other children don’t have an interest in mathematics. One day, my third daughter, who was in the “classe préparatoire” for management (about 18 years old), needed help, but with no real interest. I had great trouble in convincing her to keep her mobile off. I don’t have very nice memories of that time.
[AM/TMF]: We know that each of your children plays an instrument. What was the role of music in your family life?
CV: Yes, bassoon, oboe, viola, piano, cello. They are quite good. I started playing the violin late. I believe I don’t have a nice sound; it is just for fun. I never forced them to study, I thought it had to come from them. But I encouraged them to play an instrument because it is excellent to create discipline since one must play every day. They all ended up being good musicians.
[AM/TMF]: Is any of your children a professional musician?
CV: No… The problem is that it is hard to have a good life. You must give concerts but also teach. Leila Schneps has four children and one of them is a professional musician, quite encouraged by her, but I did not encourage mine. I think it is a very hard life. There are many disappointments, merit is not easily recognized. While at the university you can teach and do research, and you are recognized in a fairer way.
[AM/TMF]: You must have a passion for what you do… Could you say you love Paris?
CV: Yes, undoubtedly! It is true. We spent 23 years in Bourg-la-Reine, which is a very nice small town, very convenient for raising children. Eleven years ago, we moved to Paris when they were finishing high school. I feel much better in Paris where I walk a lot, thinking about mathematics. Working in Bourg-la-Reine was not as effective. In Paris, I am more focused.
[AM/TMF]: Will Paris be more exciting?
CV: It is a very intense city. The architecture, the environment, are thrilling.
[AM/TMF]: Other interests of your youth were philosophy and poetry. What is it like in the present? Can you share with us some authors of your choice?
CV: In poetry I really liked Mallarmé, Baudelaire, Rimbaud, Gérard de Nerval and the classics in general. More recent, René Char, Philippe Jaccottet. I currently don’t read poetry, but poetry left an impression on me. For example, Baudelaire stayed in my memory. As well as, in the case of philosophy, I remember for example Jean Cavaillès. Forty years ago, my list of authors was long. In fact, nowadays, my tastes have changed, I read more novels, biographies, essays, history books. I continue to read a lot.
In her office in 2022 (courtesy of Claire Voisin).
🅭🅯 CC BY 4.0[AM/TMF]: Returning to the question, we know that you did “speed dating” with young students at the Collège de France. Despite teaching not being part of your duties, do you enjoy it?
CV: It is hard to say. I don’t think I have patience to teach, especially with teenagers. Although some experiences went well. I really enjoy teaching master’s courses and I had to do it when I was at the Collège de France. In this case you must be very well organized, you must try to encourage. It is very demanding to effectively introduce often sophisticated tools necessary for research. It is not like giving a talk where sometimes you must be vague.
Students must understand all from the beginning. For me, it is very demanding, but I am interested in giving courses from time to time.
[AM/TMF]: We remarked that you don’t avoid public discussion around mathematics, giving interviews, talks, etc. In one of these, we particularly appreciated one of your statements: “Tous les puissants outils théoriques que nous développons trouveront tôt ou tard une application.” (All the powerful theoretical tools we develop will sooner or later find an application.)
CV: This takes us to the question of why. Maybe because I work in pure mathematics, particularly in algebraic geometry, where there are lots of questions we would like to answer, but the applications seem to be empty. Often, we are confronted by ourselves and others about why we do it. Honestly, I think that this question is wrong, firstly because we are deeply interested in a theme, secondly because that is the way mathematics develops.
It develops by creating tools for us, but in the future these tools will serve other purposes. I don’t believe what I do is useless.
[AM/TMF]: Of course not. We don’t ask a musician why he makes music and what it’s for.
Notes by herself (courtesy of Claire Voisin).
🅭🅯 CC BY 4.0A painting painted by herself (courtesy of Claire Voisin).
🅭🅯 CC BY 4.0CV: That is another aspect, but that is not what I am referring to. Of course, that is a good answer because it [music] is an essential activity. Human beings like abstraction. And mathematics is the only science where you find it with the greatest rigor. I think mathematics is the most important science for the development of human thinking. However, I wanted to emphasize the importance of the mathematics we are currently doing. The theorems I am proving may not have a direct application, but the tools I am developing and creating are certainly useful for the development of science.
[AM/TMF]: Arnaud Beauville, your PhD advisor, gave a talk about your proof of the Green conjecture at the famous Bourbaki Seminar in 2003. He was a senior and Claire a junior. It looks like a role reversal. How did you feel at the time?
CV: I was not that junior, when he gave that talk at Bourbaki, I was already in my forties. I no longer considered myself a junior. Still, it was very nice of him to do so. I suffer with the fact that Arnaud Beauville, despite being so famous and highly regarded in France, is not a member of the Academy. He is so strong and so good!
I don’t understand why I am and he is not. There may be a number of political reasons why he isn’t, and I got the title just because I am a woman. Nowadays, it is fine to give these titles to women. I don’t like this situation and I consider it very unfair.
[AM/TMF]: This takes us to another question. How do you deal with being the only woman who won the Shaw Prize?
CV: As a person, I always have been lonesome, and, as I said before, I mostly lived at home without socializing. In other words, I never suffered from living in a mostly male world. Eventually, if I had been more involved with university life, I might have suffered more from these conditions. Therefore, I never thought much about being the only woman. It was really a recognition. I confess that I was, and I am, a little afraid that I have been awarded the prize exactly because of that, because of being a woman. This idea for me is painful.
[AM/TMF]: We don’t believe it. The prize is yours because you deserved it!
CV: Look at the media… What they say, the only thing they say, when you receive a big award, is that you are a woman. They don’t talk about the work. And I don’t just speak for myself, I speak in general. When Karen Uhlenbeck won the Abel Prize, the only thing the newspapers referred was that she was a woman. It is absolutely dramatic that they only focus on this aspect. When you are a woman who goes through this situation, when you work, your gender does not matter. Unfortunately, we conclude that what is most important to many people is that we are women. More than our work.
[AM/TMF]: It is sad that we still must talk about this. About being women. We should already be in another stage…
CV: Sometimes I feel myself caught in a trap. I became a woman mathematician. Not simply a mathematician. I am regarded as a woman mathematician. I didn’t want it to be like this. I didn’t want the mathematics I do to be appreciated or evaluated from the point of view that I am a woman.
[AM/TMF]: Maybe this vision is persistent because you have a large family and family life usually is limiting in achieving great successes.
CV: Do you know Christopher Hacon? He works in algebraic geometry and has six children! His wife has a job. For him there is no contradiction between being an excellent mathematician and his family life.
[AM/TMF]: It is as you said: you must be focused on your work. We understood that, for you, Claire, work is more important than prizes themselves. But we can’t resist asking: what work did you most enjoy being recognized?
CV: For me, perhaps the recognition I most appreciated was the talk at the Bourbaki Seminar on my work. First, because I am proud of my work and second, because the Bourbaki Seminar is an institution in France.
I must say that, for sure, it is the best recognition I ever received. Someone you like talking about your work… Of course, receiving prizes is also very pleasant, but they don’t have the same meaning. I concede that they give us more visibility, but the latter is not so easy to manage. After prizes, after their involvement and their consequences, it becomes harder to return to our corner and to do mathematics. It is somehow disturbing.
[AM/TMF]: You have lots of students. How do you manage them? Do you give a problem to each of them? Do you follow them closely?
CV: I follow them closely, I give them problems that are in general developments of my own work, I more or less know where they go. I have my own strategies. The best students have more freedom to do what they want, and I give others lots of clues.
Nowadays, a PhD thesis takes only three years, including a report; my students must have two or three written works. I never give them completely open problems which I don’t know where they go. These three years are when they learn a lot, it is the time when they become researchers, which they learn by writing papers, by proving theorems. Usually, I go to the university at least once a week to participate in my seminar and to follow my students.
[AM/TMF]: Do you currently have any problems that you are most obsessed with at present? Could we suggest you talk a little about your work on mirror symmetry?
CV: I stopped working on that long ago. I stopped for two reasons: one is that there were already lots of people working on the subject, and the other that I didn’t keep up with the physicists. They worked fast, and I couldn’t follow with rigorous proofs. At the same time, I felt I was straying from my own path. I preferred to follow my own questions. About the other question, I now work on a subject that interests me a lot, very beautiful, the hyperkähler manifolds. They are compact Kähler manifolds which may not be algebraic, their geometry is more abstract and, in general, they don’t contain interesting complex submanifolds. Of course, we understand them better via deformation theory and come back to algebraic geometry thanks to Kodaira’s theorem: many are algebraic, many are not. A bit like complex tori and abelian varieties. The theme appeals to different areas of mathematics, like complex differential geometry, the properties of Kähler metrics and, of course, algebraic geometry. Their moduli spaces are known thanks to Torelli’s theorem. Their algebraic cycles are very particular. These varieties are a bit like abelian varieties, but more mysterious because they don’t possess a group structure.
I have been working on that for the last two or three years. We received an ERC Synergy Grant for this project. I work with Professors Debarre, Macrì and Huybrechts. The project, financed with 8.5 million euros, consists in testing some of the fundamental conjectures of modern mathematics on hyperkähler manifolds. This is a subject on which I already wrote a number of joint papers, while I usually publish alone. It is something new to me which turned out to be quite enjoyable.
I am currently investigating the algebraic cycles. I want to understand them like in topology.
We see them as subvarieties of an algebraic variety, and we introduce some relations on the generated abelian group. This is how Chow groups appear.
During the EMS awards ceremony. We recognize starting from the right: Labourie, Hirzebruch, Karoubi, Jacques Chirac, herself, Franke, Goncharov, Kontsevich (and other laureates) (courtesy of Claire Voisin).
🅭🅯 CC BY 4.0[AM/TMF]: Close to the theory of motives?
CV: Yes, a theory very much related to motives, from the complex algebraic geometry, Hodge theory and Hodge conjecture viewpoints. Chow groups are what we compute in a motive. Of course, motives are more general.
[AM/TMF]: To finish, who were the mathematicians that most influenced or inspired you?
CV: Clearly, when I started, Philip Griffiths. He influenced me a lot. He was working on Hodge theory. When I started my studies, I wrote a number of papers influenced by his work. I did not know him yet. I only made his acquaintance later, because we started a discussion on Chow groups. Fulton was also very influential. He wrote an amazing book called Intersection Theory where he formalized intersection products in the Chow groups, which were essential and valuable for solving lots of problems. This completes Griffiths’ work, who always used complex geometry. Now, in Fulton’s book, the approach is from algebraic geometry.
There are other mathematicians whom I consider as most important in my area, and who influenced me, like Jean-Pierre Serre and also Alexander Grothendieck, but I never met him.
[AM/TMF]: To conclude, Claire, is there any question you were never asked despite the fact you would like to be asked, about your life as a mathematician?
CV: In the interview proposal you sent me, there was a question I would like to answer, namely, what am I most proud of in life? I thought a lot about this question, and I would say I am most proud that I didn’t lose my passion for mathematics. In fact, I think it is the opposite: the more I work, the more I do research, the more I know my area, the more interesting my studies become. Maybe things would have been a little different when I was turning 40. It was a matter of life and death. Maybe it was too much then. Currently, I deeply like what I do. It is so interesting, there are so many questions, there are so many paths to follow! I am very happy I haven’t lost my passion!
Claire Voisin being awarded the Crafoord Prize
[AM/TMF]: Claire, this is a philosophical question: do you think this prize [the Crafoord Prize] was awarded to you at the right moment?
CV: It is difficult to answer… It is hard to say you deserve a prize. From this point of view, the sooner, the better. I don’t know if it is the right moment, but this is a most important prize, and I will do my best to be at its level and to convince people that I deserve it.
[AM/TMF]: You are already on a pedestal! Your answer seems to reveal a feminine nuance corresponding to the eternal need of women to always prove what they deserve.
CV: This is not exactly the feminine side. The question is my area, algebraic geometry, where exceptional mathematicians like Serre, Grothendieck, Deligne, affirmed themselves. They made us feel so small… It would be different in a more recent area. Algebraic geometry and Hodge theory have a long history. I can mention, for example, Riemann and the theory of hyperelliptic integrals. In the master course I teach at present, I spend lots of energy explaining the strength of these results and their extreme importance for algebraic geometry.
[AM/TMF]: How did your students react to your prize?
CV: They may not be aware of it, since they don’t talk about it. And I prefer not to be embarrassed with many demonstrations.
[AM/TMF]: Picking up this question, we know that often, mathematicians give parties when they are awarded prizes. How is it going to be?
CV: I will have a party with my children, but only after concluding the course I talked about, because it takes a lot of energy out of me.
[AM/TMF]: Does this prize embrace all your mathematical work, or does it emphasize some particular result?
CV: The prize refers to my work as a whole. Personally, I would highlight the paper on Green’s conjecture, the paper on Kodaira’s problem, but also the paper on the stable rationality of algebraic varieties by specialization methods.
Returning to the question about “the right time,” sometimes awards happen when you are depressed, in an impasse, the research is not progressing, and what you do seems to be useless, but this was not the case. I am very happy with the recent work with János Kollár which answers a problem going back to the sixties. This is an important and pleasant work, not yet published. The main result is quite easy to state, and the proof is very elegant. I include this work among my most important results.
[AM/TMF]: Will your life change?
CV: As I said before, I’ll keep working hard but not really because of the prize. Each of us has an intimate master, with one’s own orientations and criticisms.
[AM/TMF]: Do you intend to go to Sweden to receive the prize?
CV: Of course! I like to be polite. This prize represents a financial support to science.
We must be grateful and polite. Our governments, for the most part, are not very respectful where science is concerned. It’s a duty of politeness towards those who are.
Acknowledgements. The EMS Magazine thanks Gazeta de Matemática for the authorization to republish this text, which is an English translation of the original interview in Portuguese entitled “A matemática enquanto espaço privado: do desvendar de conjeturas ao reconhecimento mundial” and published in Gazeta de Matemática, 0202, pp. 44–54, March 2024.
Cite this article
Ana Isabel Mendes, Teresa Monteiro Fernandes, Claire Voisin. Eur. Math. Soc. Mag. 134 (2024), pp. 24–31
DOI 10.4171/MAG/206

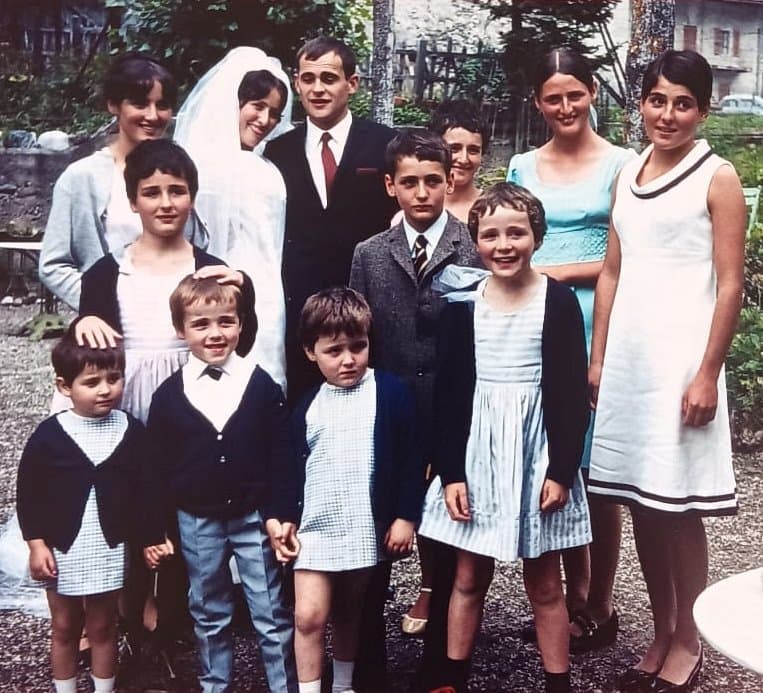
_cropped.jpg&w=3840&q=75)



_cropped.jpg&w=3840&q=75)